Unlocking Solutions: A Beginner's Guide to Linear Equations
Imagine trying to calculate the exact amount of ingredients needed to double a recipe. Or perhaps you're trying to determine how long it will take to travel a certain distance at a given speed. These everyday situations, and countless others, can be easily represented and solved using the power of linear equations.
Linear equations, sometimes referred to as first-degree equations, are the cornerstone of algebra and a fundamental concept in mathematics. They provide a powerful framework for modeling relationships between variables and solving for unknown quantities. Understanding linear equations opens doors to comprehending more complex mathematical concepts and empowers us to tackle practical problems with confidence.
The history of linear equations can be traced back thousands of years, with evidence suggesting their use in ancient civilizations like Babylon and Egypt. These early mathematicians developed ingenious methods for solving linear equations, often in the context of land surveying, construction, and astronomy. The development of algebraic notation during the Renaissance further revolutionized the study of linear equations, paving the way for modern mathematical advancements.
At their core, linear equations involve a single variable raised to the power of one. They take the general form of ax + b = c, where 'x' represents the unknown variable, 'a' and 'b' are coefficients, and 'c' is a constant. The beauty of linear equations lies in their simplicity and versatility. They enable us to model a wide array of real-world phenomena, from predicting population growth to analyzing financial data.
Mastering linear equations is essential for anyone seeking to navigate the world with a mathematical lens. Whether you're a student grappling with algebra homework or a professional seeking to make informed decisions, understanding linear equations empowers you to approach problems systematically and arrive at accurate solutions.
One of the primary advantages of linear equations is their ability to express direct relationships between variables. For instance, consider the equation y = 2x + 5. This equation tells us that for every increase of 1 unit in 'x', the value of 'y' increases by 2 units. This direct proportionality allows us to make predictions and understand how changes in one variable impact another.
Moreover, linear equations provide a structured approach to problem-solving. By representing real-world situations as linear equations, we can leverage algebraic techniques to isolate the unknown variable and determine its value. This process involves performing the same operation on both sides of the equation until the variable is isolated, adhering to the fundamental principle of equality.
Furthermore, the study of linear equations lays a solid foundation for comprehending more advanced mathematical concepts. Concepts such as systems of equations, matrices, and linear programming all stem from the fundamental principles of linear equations. A strong grasp of these basic concepts is crucial for anyone pursuing further studies in mathematics, science, engineering, or any field that involves quantitative reasoning.
While linear equations offer numerous benefits, it's essential to acknowledge their limitations. In real-world scenarios, relationships between variables are often more complex than the simple, linear relationships represented by these equations. Factors such as multiple variables, non-linear relationships, and external influences can all impact the accuracy of models based solely on linear equations.
Despite these limitations, linear equations remain an indispensable tool for understanding and modeling a wide range of phenomena. They provide a valuable starting point for analyzing relationships between variables and offer insights into the underlying dynamics of complex systems. By recognizing their strengths and limitations, we can leverage the power of linear equations to solve problems, make informed decisions, and navigate the world with a mathematical mindset.

ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica
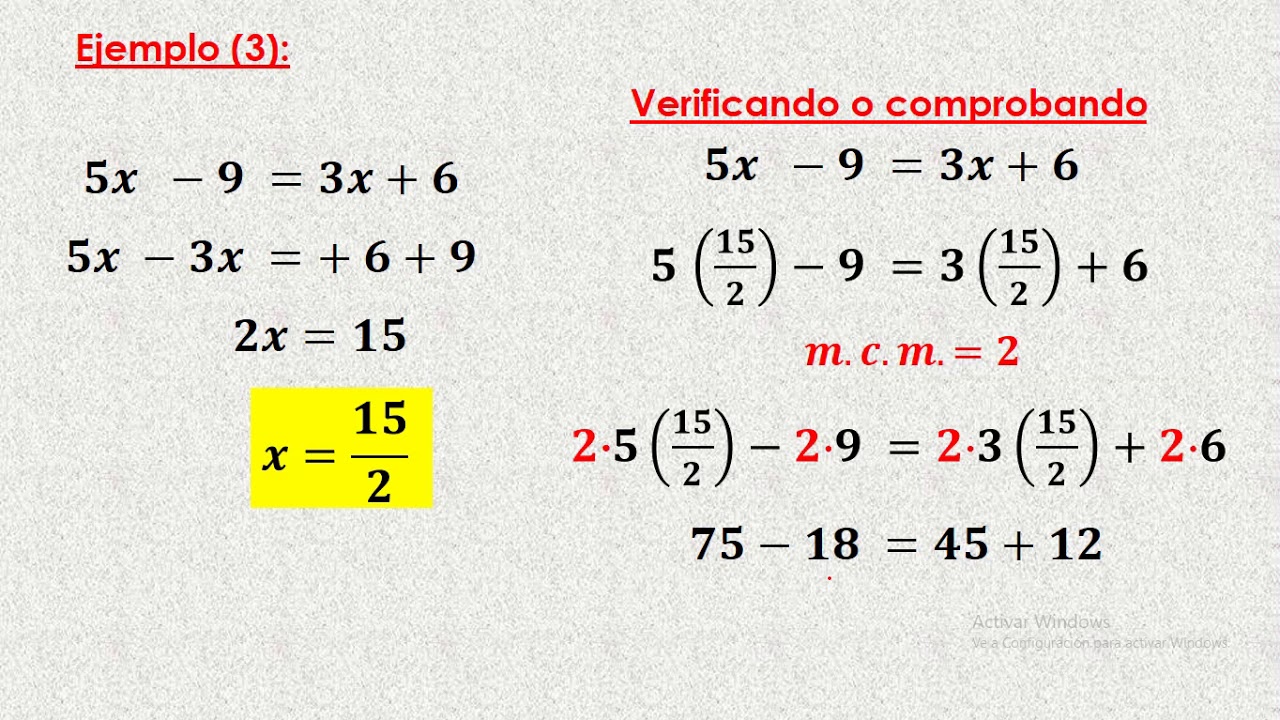
ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica

ejemplo ecuaciones de primer grado | Taqueria Autentica
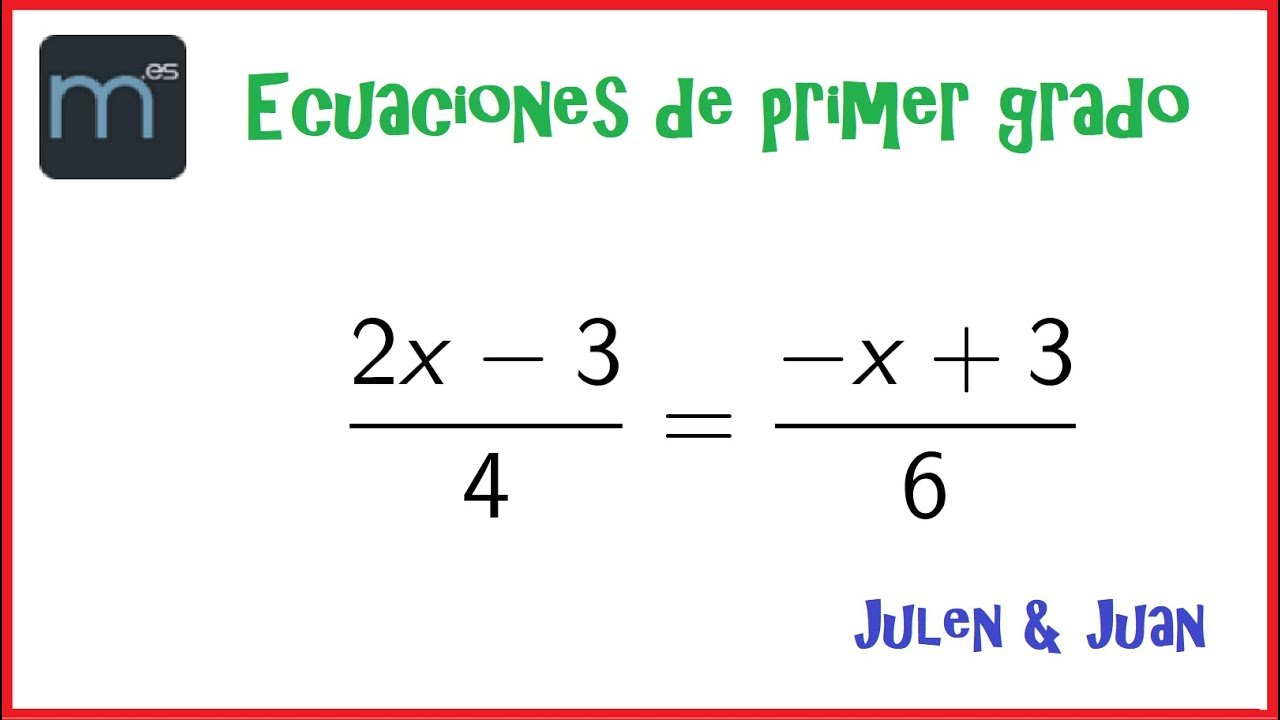
ejemplo ecuaciones de primer grado | Taqueria Autentica