Unlocking the Secrets of Triangles: A Practical Guide
Imagine gazing up at a majestic redwood, its crown seemingly kissing the clouds. You wonder, how tall could this magnificent giant be? Or picture yourself on a sailboat, charting a course using the stars and the angle of the sun. These scenarios, while seemingly worlds apart, are united by a common thread: trigonometry. This ancient branch of mathematics gives us the tools to understand and measure the world around us, from the heights of towering trees to the vast distances across oceans.
At its core, trigonometry delves into the fascinating relationships between angles and the sides of triangles. It provides us with a set of powerful functions – sine, cosine, tangent, and their reciprocals – that unlock the secrets hidden within these geometric shapes. While the formulas themselves might seem abstract, their applications are incredibly practical. Trigonometry word problems bridge the gap between theoretical knowledge and real-world scenarios, challenging us to use these functions to solve tangible problems.
The origins of trigonometry can be traced back thousands of years to the ancient civilizations of Egypt, Babylonia, and Greece. These cultures recognized the importance of triangles in fields like astronomy, architecture, and surveying. For instance, the Egyptians used a system of ropes tied at specific intervals to create right angles for their monumental pyramids. As centuries passed, mathematicians like Hipparchus and Ptolemy developed the first trigonometric tables, laying the groundwork for the sophisticated trigonometric functions we use today.
Understanding trigonometric concepts and their applications through word problems is crucial for various reasons. It enhances our spatial reasoning abilities, allowing us to visualize and analyze geometric relationships more effectively. Moreover, it provides a framework for solving practical problems in diverse fields. From engineers designing bridges and architects planning skyscrapers to physicists studying projectile motion and computer graphics designers creating realistic 3D models, trigonometry is an essential tool.
However, the journey through the world of trigonometry word problems isn't always smooth sailing. Many students struggle with translating word-based scenarios into accurate diagrams, identifying the relevant trigonometric ratios to use, and solving equations involving trigonometric functions. This is where a structured approach, clear explanations, and ample practice become vital. By breaking down complex problems into manageable steps, mastering the underlying concepts, and developing problem-solving strategies, anyone can conquer the challenges of trigonometry word problems.
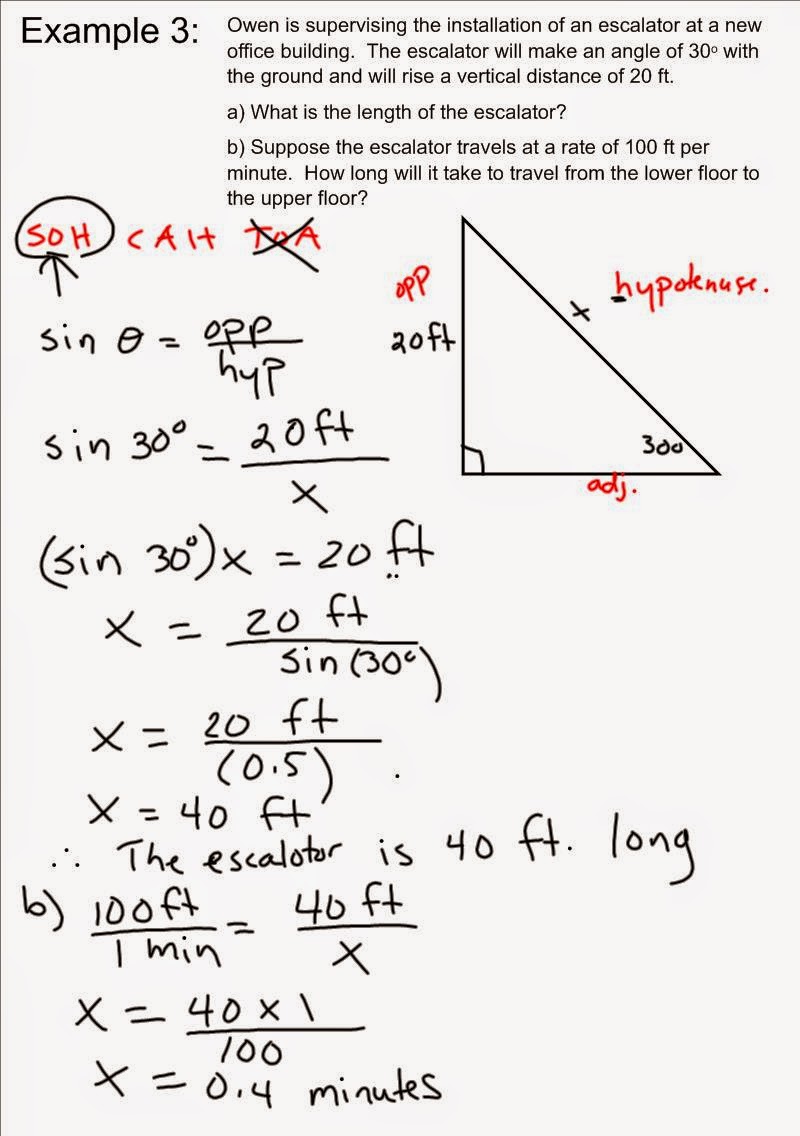
Let's consider a simple example to illustrate the power of trigonometry in action. Suppose you're standing 100 feet away from the base of a tall building, and the angle of elevation to the top of the building is 30 degrees. How tall is the building? By applying the tangent function (tangent of an angle = opposite side / adjacent side), we can set up an equation: tan(30°) = height / 100 feet. Solving for the height gives us approximately 57.7 feet. This simple example demonstrates how trigonometry helps us find unknown measurements using readily available information.
Advantages and Disadvantages of Tackling Trigonometry Word Problems
| Advantages | Disadvantages |
|---|---|
| Enhances problem-solving skills | Can be challenging to translate word problems into mathematical equations |
| Develops spatial reasoning and visualization abilities | Requires a strong understanding of trigonometric concepts and formulas |
| Applicable to real-world scenarios in various fields | May involve complex calculations and algebraic manipulations |
Mastering trigonometry word problems is a journey that requires dedication and practice. But with the right approach, anyone can unlock the power of this fascinating branch of mathematics and apply it to understand and solve problems in the world around them.

trigonometry word problems with solutions | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica

Law Of Cosines Worksheets | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica

trigonometry word problems with solutions | Taqueria Autentica
trigonometry word problems with solutions | Taqueria Autentica